A Sigmoid No Threshold Primer

If we are to replace the Linear No Threshold theory of radiation harm, we must have a replacement. The purpose of this piece is to present the Sigmoid No Threshold dose-harm model and compare it with LNT. The piece requires a bit of background, at a minimum reading LNT is Nonsense. You will need to know that dose, the amount of radiation energy deposited in a kilogram of tissue, is measured in milligrays (mGy).

Two Acute Dose-Harm Models

The overriding safety concern about nuclear power is the health hazard associated with a release of radioactive material. We have been told over and over that any such release is a catastrophe. But we live in a sea of radiation. Depending on where you are reading this, in the last minute your body has absorbed between 1 and 10 million particles with enough energy to produce cell damage. Life evolved in an environment where the natural level of radiation was 5 times higher than it is now. If radiation is so harmful, why are we here?

The answer is life evolved a system, an extraordinarily clever system, for handling this onslaught. The system is so automatic that we are unaware of it. For many hazards, evolution developed sensors and responses, so we can react to a danger. Too much heat will destroy tissue. So we developed nerves that sense temperature and send a signal called pain to the central nervous system that tells us "stop touching, get away". But there's no getting away from radiation. So evolution went with a system that repairs radiation damage without our needing to do anything.

At the cellular level, these systems work on time-scales of minutes to about ten hours. At the organism level, the repair period is a few days depending on the dose rate. Doses which are received within a single repair period are known as acute doses. Doses that are spread over multiple repair periods are called chronic doses.

Our repair systems can be overwhelmed if the dose rate is high enough. One way to create such dose rates is to drop an atom bomb on people, as we did at Hiroshima and Nagasaki. At great expense, the doses of 80,000 survivor has been estimated by the Radiation Effects Research Foundation (RERF), funded by the US and Japanese governments. Since almost all these doses was received in a matter of minutes or less, these were all acute doses.

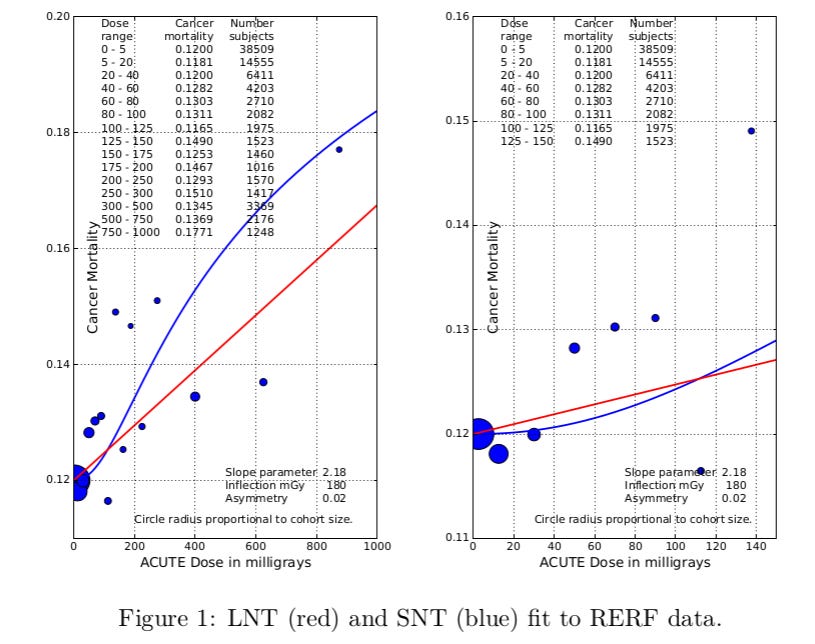
Two competing dose-harm curves have been fitted to this acute dose data.

1) Linear No Threshold (LNT) LNT is based on fitting a straight line through the data,

2) Sigmoid No Threshold (SNT) SNT is based on fitting an S-shaped logistic curve to the data. This is conventional dose-response practice outside of radiation.

Figure 1 compares these two fits. The RERF cancer mortality data bounces up and down in an inexplicable manner, making it impossible for any function to yield a good fit. In a nuclear power plant release, the doses to the public are almost always at the very low end of the bomb survivor range, the right panel in Figure 1. The SNT fit is clearly better at the very low end. In that range, the absolute difference in harm is small, but the relative difference can be very large, and increases very rapidly as the dose decreases.

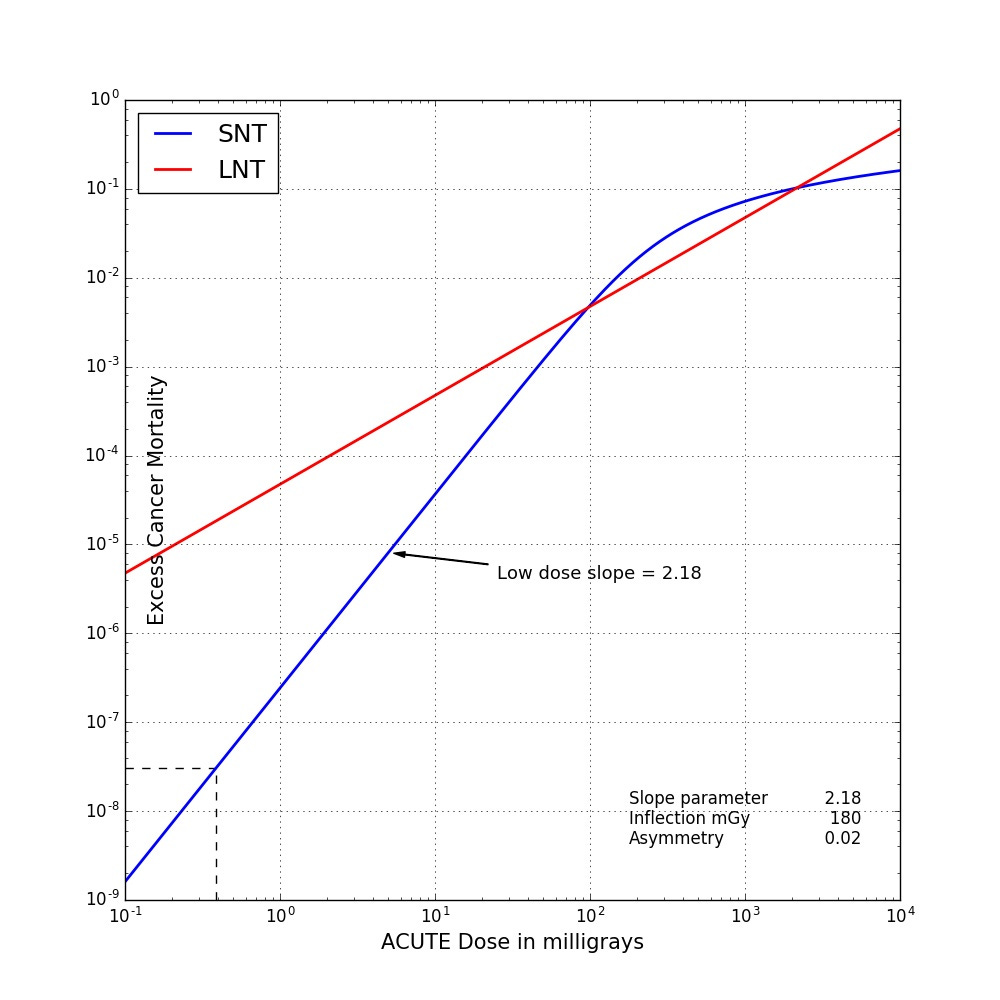
To see this, we need a log-log plot, Figure 2.

Figure 2. LNT (red) and SNT (blue) fit to RERF data, log-log plot.

This figure shows that, while the SNT fit is above the LNT fit in the 200 mGy to 2000 mGy range, below about 100 mGy, the fits diverge rapidly. At 0.1 mGy, the curves differ by a factor of 6000. Figure 2 also makes the point that, at the low end, the logistic becomes a power law in which the exponent is the slope parameter, in this case 2.18. LNT is also a power law whose exponent is 1.0.

But power plant release doses are chronic?

In a nuclear power plant release, the public doses are almost always received over an extended period of time: weeks and months and years. How can we use our acute dose-harm models to model the harm associated with chronic doses?

One possibility is to pick a repair period; and treat the dose within each repair period as if were acute. At the end of each repair period, we will have some unrepaired damage (aka harm) depending on our acute dose harm model. We then add up the individual repair period harms, effectively assuming the repair period harms are independent. The idea is either the repair process works or it does not. If it's the latter we are going to get cancer.

To have a concrete example to work with, consider a situation where someone is exposed to 10 mGy/d for 4 weeks, and we assume a repair period of a week. We can represent the unrepaired damage at the end of each repair period by an arrow.

If our dose-harm model is LNT, we have the harm profile shown in Figure 3. We notice something peculiar. When we add up the individual repair period harms, we end up with the same harm as if the entire dose was acute, as if there were no repair, the big red arrow.

Figure 3. Dose rate 10 mG/y for 4 weeks. Repair period = 1 week. LNT harm.

In fact, if you think about it, if the dose-harm is linear, how ever you chop the dose-profile into repair periods, when you add up the repair period harms, you will come up with the same number. The fancy word for this is superposition. In plain language, a linear harm model implies no repair.

(The converse is also true. If the only thing that counts is cumulative dose regardless of how slowly or quickly the dose is received, then the dose-harm curve must be linear. Linearity and cumulative are not separate assumptions. Each implies the other.)

For LNT, the time dimension is irrelevant. It does not matter whether you get your 1000 mGy in a week, or spread over 50 years. When you add up the 2600 weekly 0.385 mGy harms, you will come up with the same total harm as a single 1000 mGy harm. In either case, LNT says your increased cancer mortality is 0.05. But that is not what happens in the real world. Not even close.

In fact, 1000 milligrays (mGy) received in a few hours will make you very sick. Vomiting, diarrhea. You may lose some hair. Your immune system will be depressed for several weeks. You will almost certainly recover; but your chances of contracting cancer sometime in the future will be increased by roughly 10%.

1000 mGy received evenly over 50 years is a dose rate of 0.05 mGy/d There are sections of the Kerala coast near Kollam where the natural background dose rate is about 0.2 mGy/d. Nearby districts have natural dose rates of 0.01 mGy/d or less. 117,000 people who live in the region were studied for 15 years. The people who lived in the high dose rate spots, people who received over 500 mGy in that period, had slightly lower cancer rates than the people who live in the low dose rate spots, people who had received less than 50 mGy. Why Nuclear Power has been a Flop lists example after example of large doses received over extended periods with no detectable harm. We start to see detectable harm only when dose rates get above 20 mGy per day.

There is no way LNT can model both the harm associated with a large dose received over a short period and the lack of harm observed when the same dose is received over an extended period. This is inherent in its denial of repair of radiation damage.

If our dose-harm model is non-linear, for example, Sigmoid No Threshold, we get an entirely different behavior, Figure 4.

Figure 4. Dose rate 10 mG/y for 4 weeks. Repair period = 1 week. SNT harm.

At an acute dose of 280 mGy, our SNT harm --- an increased cancer mortality of 0.0254 --- is almost double the LNT harm at this dose, 0.0140. (Figure 3 and 4 vertical scales are different.) But the repair period harm for a 70 mGy dose is 0.0024. When we add up the four repair period harms, we end up the a total harm of 0.0096. A non-linear dose-harm combined with a repair period allows us to bring the time dimension and our repair processes into the picture.

SNT is consistent with the fact that 1000 mGy in a hour or two creates an entirely different amount of harm than a 1000 mGy spread evenly over 50 years. According to SNT, 1000 mGy acute results in an increased cancer mortality of 0.064, a bit more than LNT. But the increased cancer mortality associated with 0.385 mGy in a week is 0.0000000267. See dashed lines in Figure 2. 2600 weeks of this results in an increased cancer mortality of 0.00007, which would be undetectable. SNT can model both the harm associated with a large dose received over a short period and the lack of detectable harm when the same dose is received over an extended period.

SNT avoids the preposterous inconsistency of claiming LNT is a realistic model of radiation harm; but, at the same time, denying we can use it to measure radiation harm in an actual release. The WHO among others adopts this nonsensical position. Take the Chernobyl release for example. Large parts of the Northern Hemisphere were exposed to slightly elevated doses.

The Union of Concerned Scientists does LNT right. Table 1 compares their analysis of public cancers due to Chernobyl with an SNT analysis using the UCS dose numbers. UCS's estimate is 21,018 statistical deaths. Nearly 80% of these deaths resulted from dose rates that are well below background in large parts of the planet. WHO and most of the radiation protection establishment say simply ignore those 17,000 deaths predicted by LNT. Is there a clearer admission that our model is hopelessly wrong?

In contrast, SNT allows us to compute everybody's harm, and ends up agreeing with the WHO et al that the focus should be completely on the people who lived in the vicinity of the plant.

Primum non nocere.

I'd be the last to claim that Sigmoid No Threshold is an accurate model of the exceedingly complex biology that is involved in radiation damage and repair. But the competition here is not perfection but LNT. Table 2 summarizes the score in that contest.

It is the last two rows that should concern the supporters of LNT. At both Chernobyl and Fukushima, the mental and physical stress caused by fear of radiation far outweighed the increase in cancer caused by the release. At Fukushima, over 1600 people were killed unnecessarily. Much of this must be laid at the feet of LNT and its promoters. These promoters have seen the human suffering and death that LNT has caused at least twice. They know that LNT is not consistent with either our current understanding of radiation damage and repair nor cancer incidence in high background dose rate areas. That why they walk away from it at Chernobyl.

If there is a far more realistic alternative that avoids these critical defects and they choose not to support it, they must share responsibility in the unnecessary suffering that will occur in the next release.